LAMI'S THEOREM

OVERVIEW
Lami’s theorem is a very useful principle that is used while performing static analysis for mechanical and structural systems.
This theorem is formulated by Professor Bernard Lamy, a French mathematician.

CONCEPT
Consider an object on which three forces are acting – force A, force B, and force C. The angle directly opposite to force A is Alpha, the angle opposite to B is Beta, and the angle opposite to C is Gamma. According to Lami’s theorem, force A is proportional to the sine of the angle directly opposite to it, which is Alpha. In other words, A is proportional to Sine Alpha. Similarly, B is proportional to sine Beta, and C is proportional to sine Gamma.

THEOREM STATEMENT
Lami’s theorem states that:
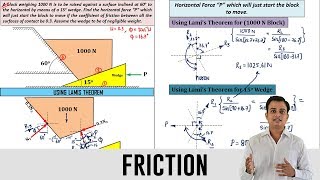
If 3 coplanar, concurrent and non-collinear forces act upon an object, and the object remains in static equilibrium, then, “A” by sine alpha is equal to “B” by sine beta, that is equal to “C” by sine gamma, where A, B, and C are the numerical values of the 3 forces and a, ß, and ? are the angles directly opposite to A, B and C respectively.

REAL-WORLD EXAMPLES
Example 1:
Consider a crane, that is carrying a load. The weight of the load pulls it downwards. A compression force acts along the JIB. A tension force acts along the tie rod. You can use Lami’s theorem, to calculate the unknown forces and angles.

Example 2:
Consider a light assembly that is suspended from the ceiling of a room. Assume that the light assembly is tied to the wall using a horizontal string. In this case, the weight of the light assembly pulls it downwards. A horizontal load acts across the string that ties the light assembly to the wall. Similarly, a load acts along the wire on which the light assembly is suspended. You can use Lami’s theorem, to calculate the unknown forces and angles.

LIMITATIONS
1. Lami’s theorem is applicable only where there are exactly 3 forces acting on the object. You cannot apply this theorem if the number of forces is more than 3 or less than 3.
2. Lami’s theorem is only applicable for co-planar forces. Co-planar forces are the forces that act along the same plane.
3. Lami’s thorem is only applicable for concurrent forces, that is, the forces that pass through a same point. You can see that, the 3 forces shown in the figure, are passing through a single point. So, these forces are concurrent forces.
4. Lami’s theorem is applicable for non-collinear forces only. Non-collinear forces are the forces that do not act across the same line. The 3 forces in the figure are non-collinear – they act across different lines.
5. Lamis’ theorem is applicable only if the object, on which the forces are acting, is in static equilibrium. On other words, the object must be balanced by the three forces and must not be in motion.

MUSIC
http://www.bensound.com/royalty-free-...

[GE6253 - ENGINEERING MECHANICS - BASICS AND STATICS OF PARTICLES]